Do your students become confused with negative base numbers and parenthesis when solving order of operation problems that include exponents? These are common challenges seen as students attempt to solve order of operation problems. Continue to discover strategies that may increase student confidence with these problems.
Negative Base Numbers with Exponents and Expressions: How to Explain
#1 – Review Positive Exponent Operations
How often have you observed students multiplying the base number of seven with an exponent of two by multiplying the base by the exponent value such as 7 x 2, vs. 7 x 7? Countless times I have also observed students attempting long division with root problems. Yes. Provide a brief overview of exponents and roots before diving into
Before introducing negative base numbers, use the following strategy to simplify positive bases with positive exponents. Using colors to help students best visualize the base (blue) and the exponent (red) in a problem such as six squared. Next, simplify by drawing two red lines, representing the exponent with a multiplication sign in between as illustrated below.

OTHER EXAMPLES
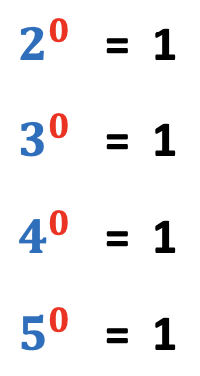



Furthermore, it is ideal for students to memorize the first twelve to fifteen values of squares, and cubes.
Also, point out that some of the square and cube roots “contain” the answer in the root. As students see this relationship, memorizing square and cube roots may become easier.
Below, notice that the square root of 25 simplified is five. The answer is in the problem. Notice this also occurs with the square root of thirty-six.
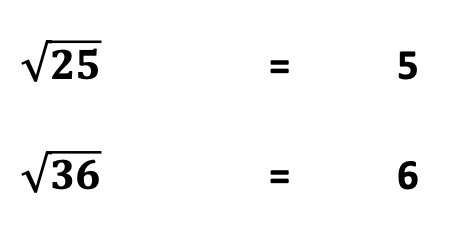
In addition, look at the cubed root below. What happens when three and four are added together? Seven! The cubed root of 512 is similar. When the digits are added together, the result is eight. Eight cubed results in the number 512.

The resource, “Introduction to Exponents and Roots,” link listed below provides additional resources to make this review easy!
Click on the link below to discover more about this resource.
#2 – Exponent Operations Using Negative Bases, No Parentheses
Next, introduce students to a negative number with an exponent with no parenthesis. This is likely the easiest rule to remember.
When the base number is negative the final answer will always be negative. See the examples below.
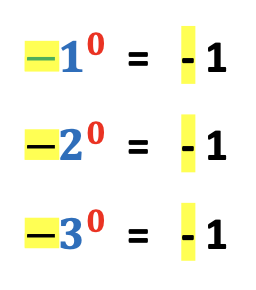
Below are more examples with negative bases and exponents of 1, 2, 3, 4, and 5. Notice that the result is always negative.

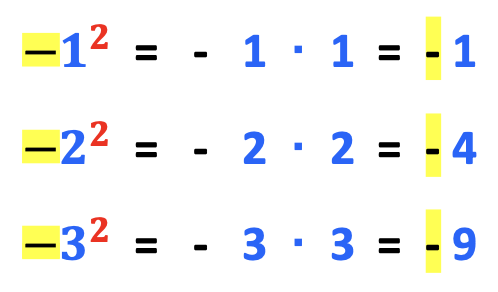
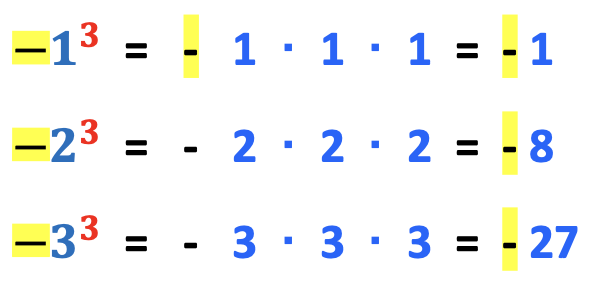
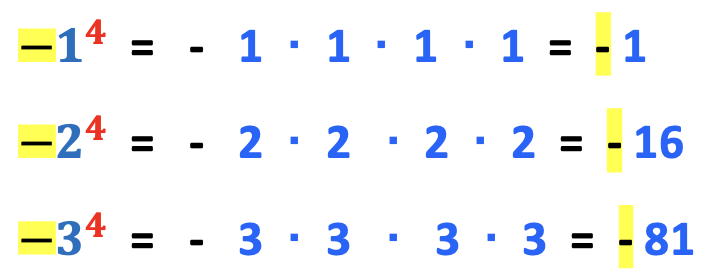

#3 – Exponent Operations Using Negative Numbers With Parentheses
On the other hand, if the base is negative and inside a parenthesis, there are two potential outcomes.
If the exponent is an even number, the result will be positive. If the exponent is an odd number, the result will be negative. See the examples below.
Examples of Exponents with Even Numbers: The result is positive.

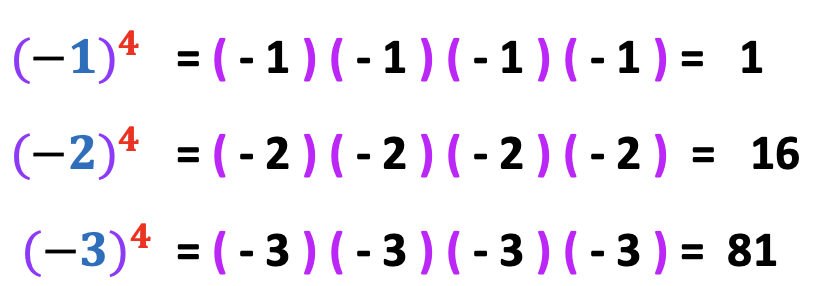
Examples of Exponents with Odd Numbers: The result is negative.
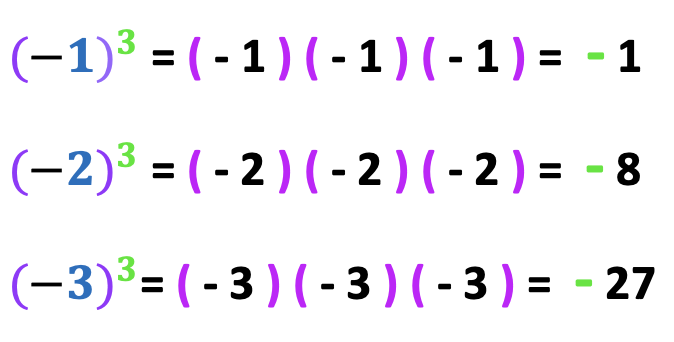

#4 – Order of Operation Problems with a Negative Base
Now let’s look at problems with negative base numbers within an order of operation problem.
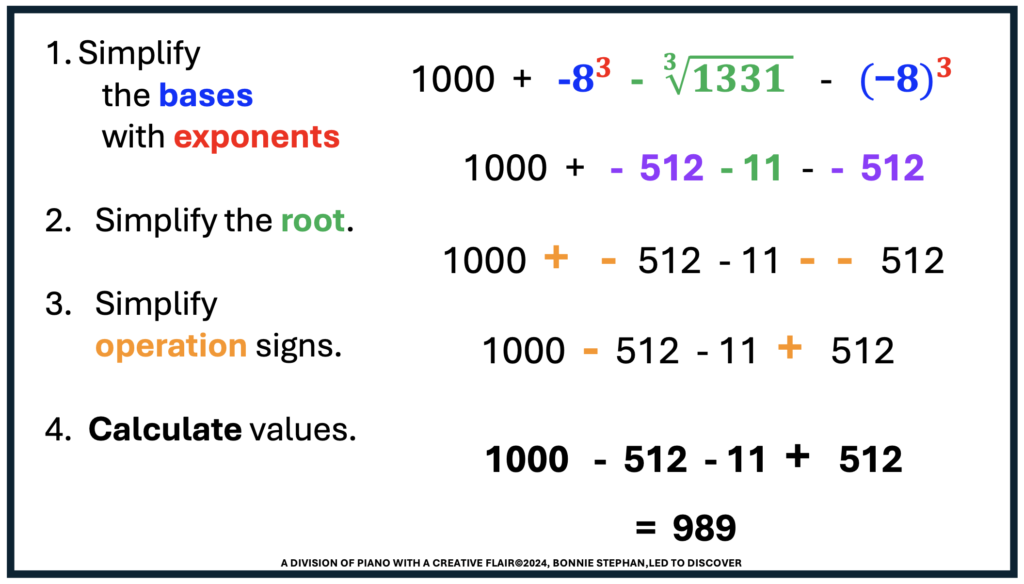
Conclusion
Students will be successfully prepared to complete order of operation problems with exponents and roots when they are confident simplifying positive and negative base numbers with exponents.
Click on the link below to discover a post related to exponents and roots.
Looking for More Materials?
Click the button below to view more of my educational resources!
