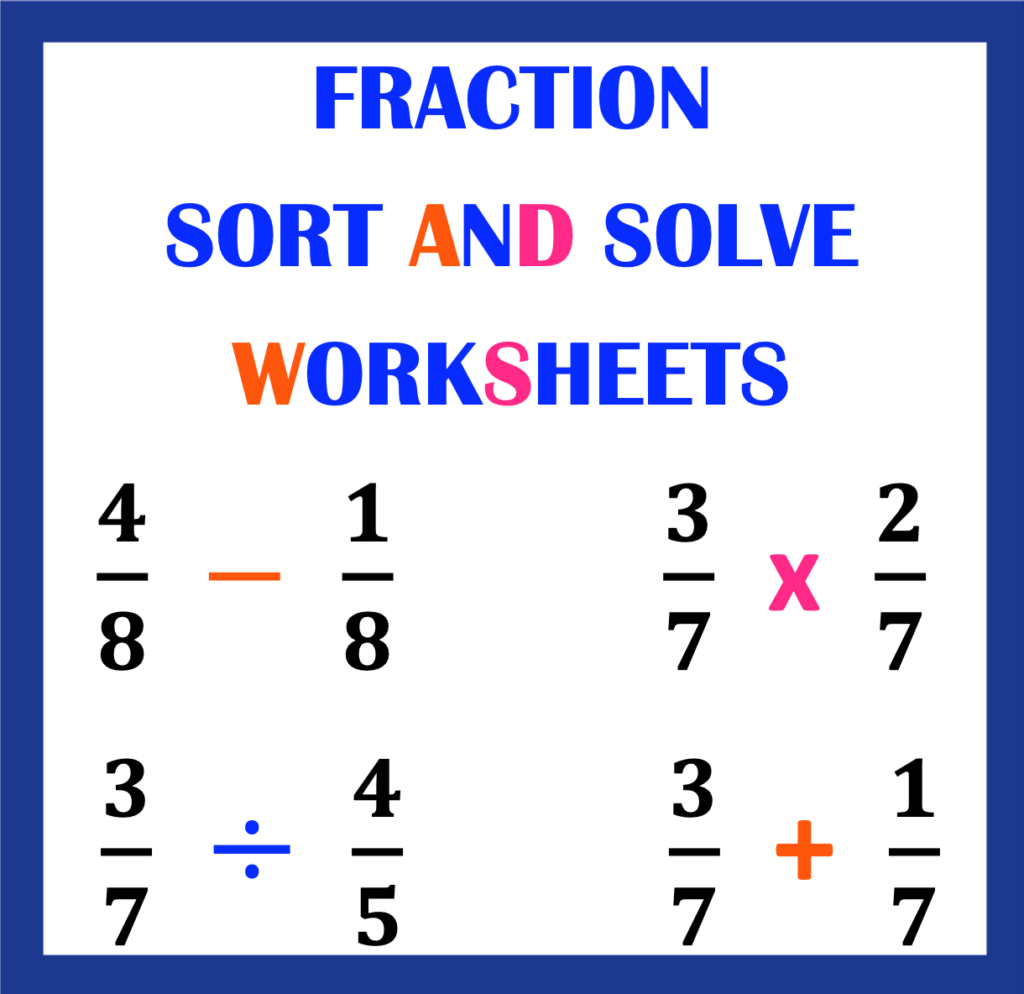
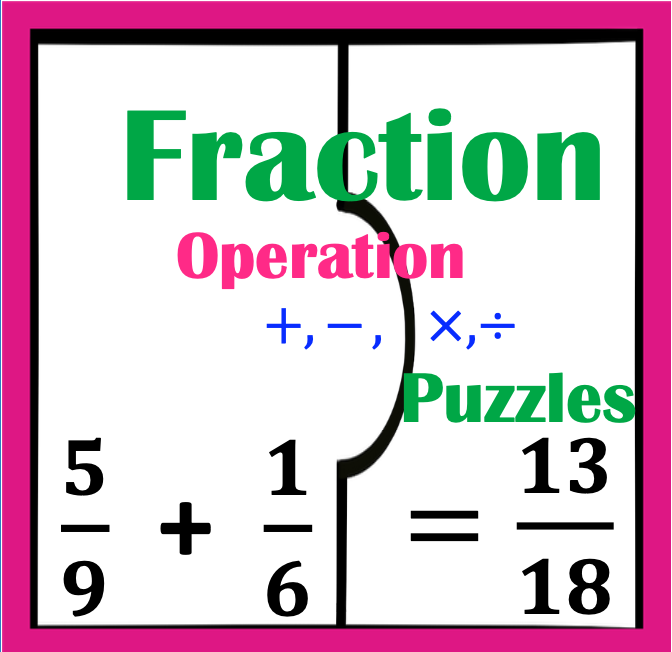
Are you looking for a way to be ready to complete order operations successfully for Algebra? This post will cover specific strategies that will successfully prepare students for order of operations in Algebra.
- 1. Why Begin with a Review of Integer Operations?
2. Increase the Number of Terms
A. Preparation for the Predictable Question
3. Three Problems, Two Operations
4. Review Absolute Value
5. Review Roots and Exponents
A. Memorize Common Roots and Exponents
B. Easy Method for Memorizing Cube Roots
6. Negative Numbers with Exponents
7. Challenge Problems
1. Why Begin with a Review of Integer Operations?
The ability to successfully complete integer operations are key for success with order of operations.
On the other hand, I have seen some materials that introduce simple order of operations that do not include negative numbers. For example, students may be given more than two or three numbers to calculate. See below.
9 + 4 – 5 + 1 + 2
3 x 2 x 4 + 1 – 5
Though I am not opposed to this, it is ideal to begin with integer operations particularly if students are in pre-algebra, as the ability to complete integer operations do indeed provide much success solving future, more complex expressions.



2. Increase the Number of Terms
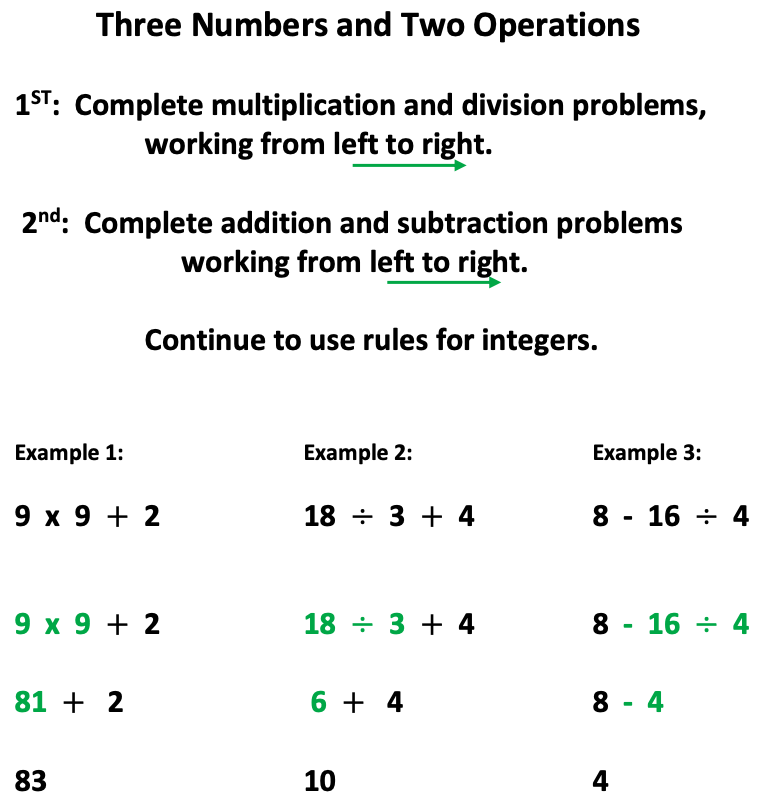
Another strategy includes providing students with practice with problems that include three to five numbers in each problem. Such problems may be grouped as addition and subtraction or multiplication and division. If the four operations are mixed, be sure to remind students to complete these types of problems in the following order.
First: Solve all multiplication and division, working from left to right.
Second: Solve the remaining expression by addition and subtraction, working from left to right.
During this practice, I insist that students solve only two problems at a time. I also insist that they skip a space between steps. As you review examples of expressions in this post, this process will be illustrated.
Below is a general recommendation for easing students into solving longer, more complex expressions which will successfully prepare students for success with order of operations.
- 1. Three to five numbers with addition and subtraction.
2. Three to five numbers with multiplication
3. Three to five numbers with division
This form of practice is to help students become comfortable solving the expression from left to right.
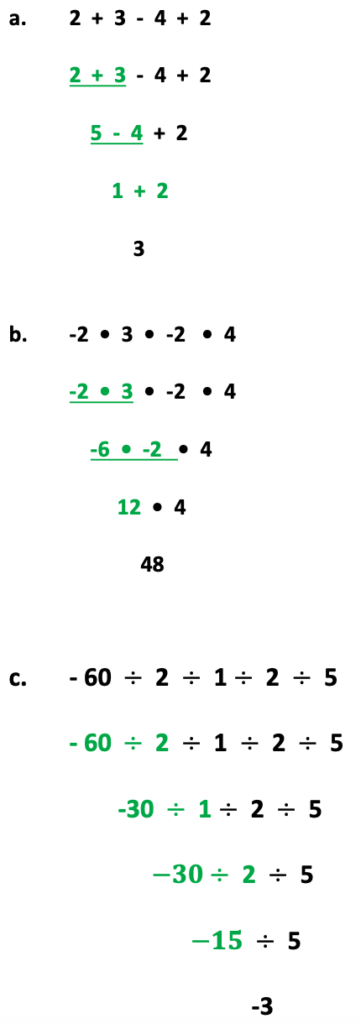
A. Preparation for the Predictable Question
“Why do I have to solve only two problems at a time when I can do it in my head?”
Of which I respond with,
“I am very impressed with your calculation ability, skills you have learned well. I will remind you that you are entering the great world of Algebra upon which steps are everything. In Algebra it is not as much about what the final answer is, but how you arrived at the answer.
So, yes. I do want you to show your steps as I am doing them on the board.”
On the other hand, depending on the types and level of complexity of the problem, as I observe student accuracy with order of operations, I may allow my human calculators to buzz along through problems, using mental math skills. At the same time, I will often provide more challenging expressions by choosing materials that include fractions and more operations wrapped up in one problem.
If students do not show steps, it is not unusual for students to demonstrate challenges and frustration upon arriving at more challenging problems. Based on this, I tend to keep my students listing specific steps for quite a while until I am convinced that they fully understand what is happening between steps. This is a lot easier than going backwards.
For this same reasons, I do not issue out calculators for students to solve expressions. The big idea of preparing students for order of operations is to ensure that they understand how to simplify various types of numbers.
3. Three Problems, Two Operations
As students demonstrate confidence working from left to right, while solving two problems at a time, I provide problems with three numbers that will require multiplication or division, and addition or subtraction. To provide support for my students who struggle, I provide color-coded problems that highlight the first two digits to solve. Next, the same types of problems are included, though without color-coding.

4. Review Absolute Value
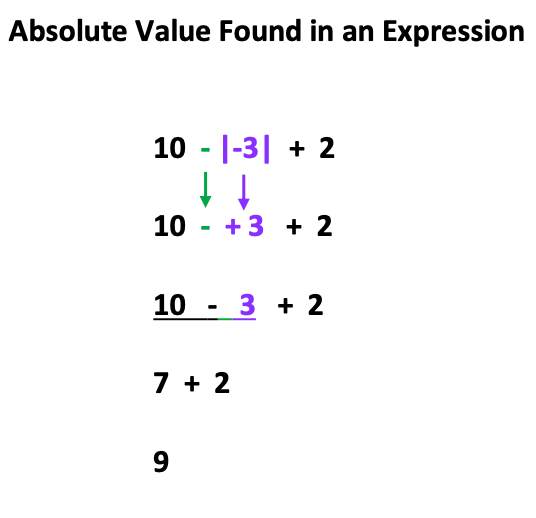
Furthermore, absolute value must also be reviewed. Numbers inside absolute value signs can be found in expressions when solving order of operation problems.
Remind students that the absolute value is the distance from zero on a number line and that distance cannot be negative.
Also, ensure that students understand how to solve absolute value when there is a negative to the left of the absolute value. This is a concept that students often have questions about while in the midst of a more complex order of operations problem. Below you will find illustrations that may be used to explain the process of simplifying absolute value as a single term and within an expression.

5. Review Roots and Exponents
A. Memorize Common Roots and Exponents
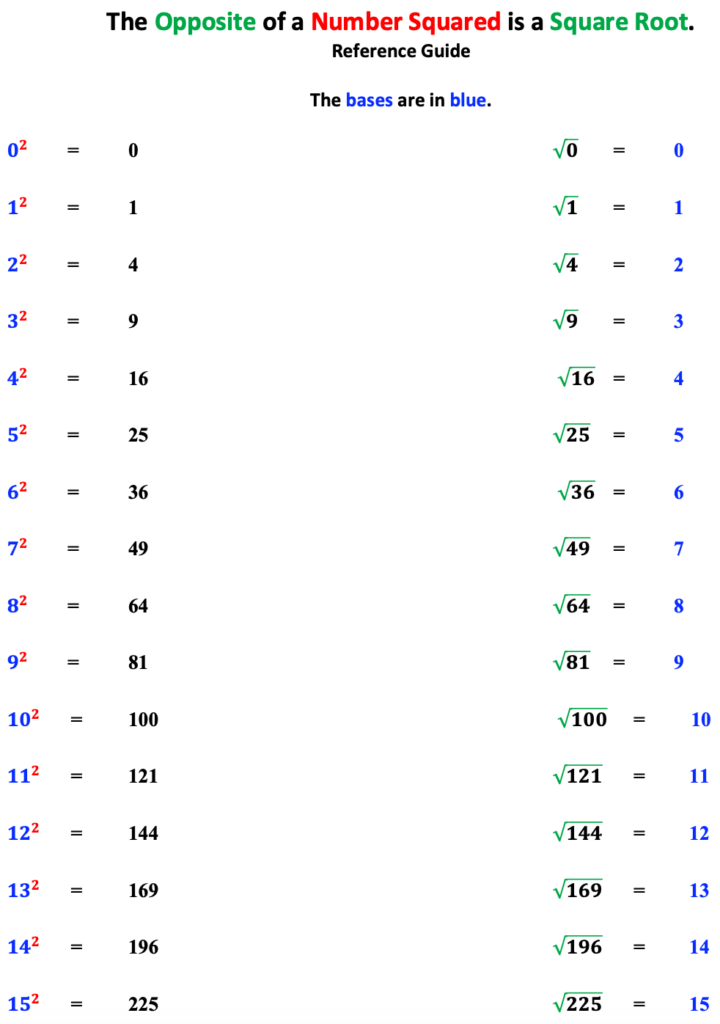
Solving roots and exponents is another key skill to learn in preparation for the order of operations. To increase math fluency, I require my students to memorize square roots from 0 to 15, and cube roots from 0 to 11.
In addition, provide examples that illustrate roots and exponents are opposites. This can be explained as illustrated below.

B. Easy Method for Memorizing Cube Roots
Memorizing the cube roots may be easier than square roots. See the number associations that can be found.

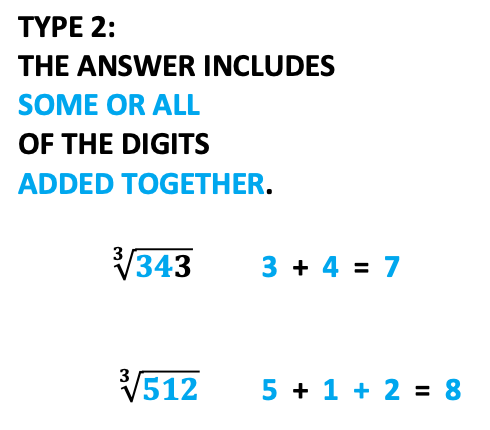

6. Negative Numbers with Exponents
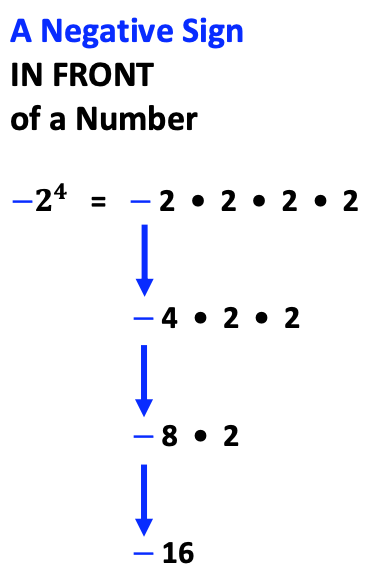
If you have taught Algebra 1, you may be very familiar with the confusion students often encounter with negative numbers with exponents.
Errors or confusion often occur with negative numbers which are within a parenthesis or outside a parenthesis. It is not unusual for these types of numbers to be found in an order of operation problem. See the examples below.

7. Challenge Problems
Some students will need more challenge. Provide these students with practice that includes:
1. Expressions with more than three digits or other types of terms
2. Absolute Value
3. Exponents greater than three
4. Roots greater than three
5. Fractions
6. Decimals
7. Word Problems
Maybe you have noticed that students do great with order of operations, but toss in some fractions with mixed numbers and unlike denominators and you will have discovered an opportunity for growth!
Other Activities to Challenge:
1. Students write their own expressions, and solve. Next, students exchange their problems with each other to determine if the answer matches.
2. Students write their own math expressions. Next, students create a word problem that could describe the math expression written.
3. Word Problem worksheets, particularly those with integers, exponents, decimals, fractions, absolute value and graphs.
Conclusion
Student success with order of operations results from a review of integers, practice with three to four numbers, absolute value, exponents, roots, and negative numbers with exponents inside and outside of the parenthesis. Students in a Pre-algebra or Algebra 1 class may present with differing abilities. To maintain class engagement, students who are competent in these topics may be provided with more challenging assignments.
Below is a packet that may be used for a review of exponents and roots that is located at my TPT store. Just download, print and go! Click on the link below.
https://www.teacherspayteachers.com/Store/Bonnie-Stephan