Research: How Important are Fractions, Decimals and Percents

Fractions, decimals, and percents: Are they as important as I think? Over the past year in previous posts, I have described concerns related to student challenges with fractions and the connections fractions have with other math subjects taught in high school. These concerns originate from my experience working with students from middle school through college-level math. In this post, I will share findings from other resources sharing similar findings. Keep reading to discover what research on rational numbers (fractions, decimals, and percents) revealed.

#1 – Fractions, Decimals and Percents: Foundations

A. My Observations: Fractions, Decimals, and Percents

When new students come for their first visit, I evaluate what they know and do not know. This seems pretty logical. Why? Very often the challenges students are struggling with are related to concepts they did not understand in previous levels of mathematics such as fractions, decimals, and percents.

For example, students who struggle with algebra concepts such as solving expressions, equations, graphing, probability, ratio, and proportions also demonstrate challenges with fraction operations.

Furthermore, I wondered if there was any research I could use to back up my experiences.

Following is a description of my research journey.

B. NCTM: National Council of Teachers of Mathematics

I began with an easy read, an article written by Francis (Skip), Fennell, the NCTM President from 2006-2008. The article was posted in the NCTM New Bulletin, in December 2007.

In this article, Fennell shares that though Pre-K through 8th grade should provide students with a solid foundation for math tasks with whole numbers, he also adds that fractions are just as important. He continues to add,

“So why am I on this fractions kick? Well, I’ve come to this position because of the following experiences:

Virtually every time I ask teachers of algebra what they wish their incoming students knew, their response is ‘fractions.'”

He also adds that his observations correspond with the National Survey of Algebra Teachers compiled by the National Opinion Research Center at the University of Chicago for the National Mathematics Advisory Panel of the U.S. Department of Education. Information regarding part of this survey is mentioned below.

C. The National Survey of Algebra Teachers

In this survey, teachers were asked the following questions:

ST U D E N T P R E P A R A T I O N

Research Question #1:

How do the teachers rate the preparation of students coming into their
Algebra I classes?

Are there widespread problems, or are problems confined to individual students?

The teachers rated students’ background preparation for Algebra I as “weak.”

In addition, they shared three skill areas in which students have the poorest preparation. These included,

“rational numbers, word problems, and study habits (Table 7).”

https://www.researchgate.net/publication/228513219_Final_report_on_the_national_survey_of_algebra_teachers_for_the_National_Math_Panel

Furthermore, I could echo the same response. Students who understand rational numbers have a greater chance of success in Algebra than those who do not.

Fennell also adds:

“Proficiency with fractions is an important foundation for learning more advanced

mathematics.

Fractions are a student’s first introduction to abstraction in mathematics and, as such,

fractions are a student’s first introduction to provide the best introduction to algebra

in the elementary and middle school years.

If you teach Algebra, I can imagine you, responding with a resounding, “Yes!”

Looking for age-appropriate fraction, decimal, and percent practice for students in high school? Click on the link below to discover practice pages that can be given daily, or weekly for continuous review.

Fractions, Decimals, and Percents Practice for High School Review

https://www.teacherspayteachers.com/Product/Fractions-Decimals-and-Percents-Practice-for-High-School-Review-11547492

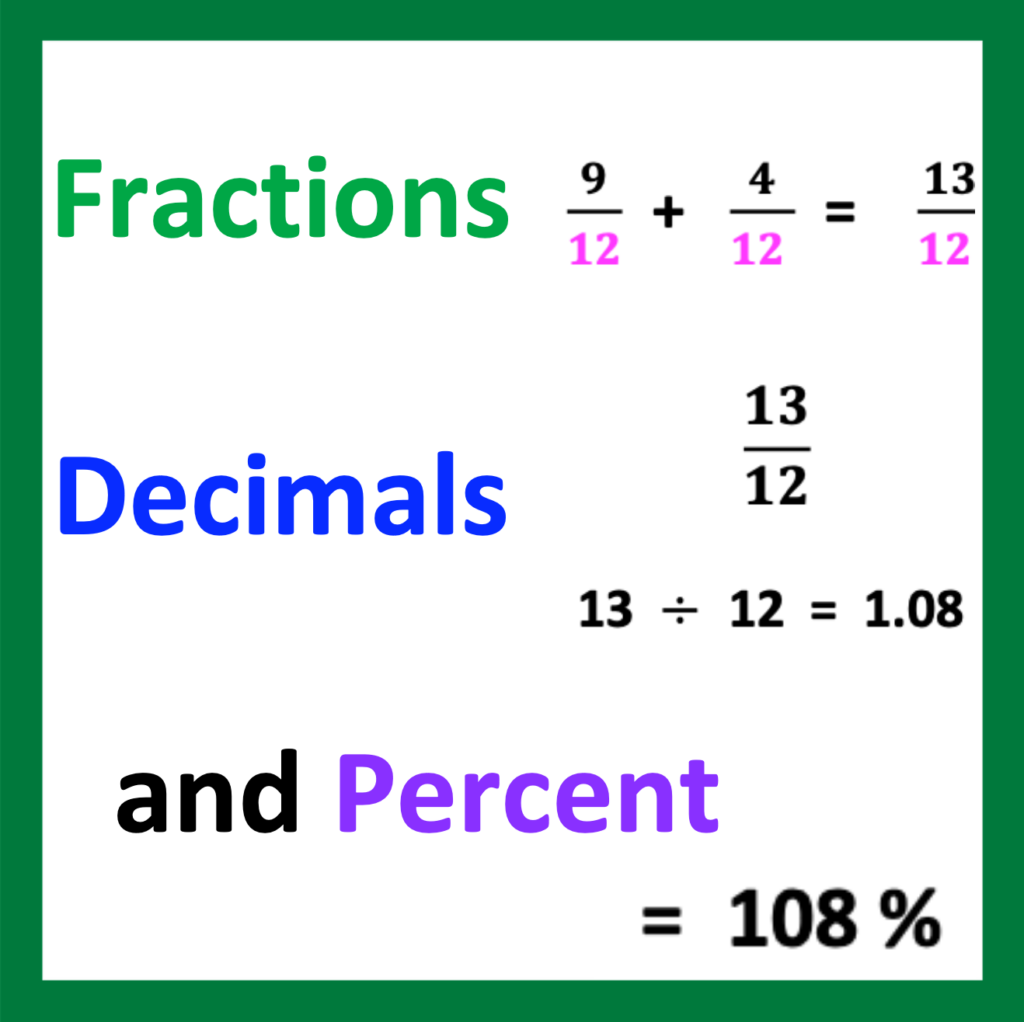
D. Rational Numbers: A Need for Time and Emphasis

In addition, Fennell continues to explain the importance of duration and intensity related to increasing student confidence with fractions.

“Time and emphasis are necessary for students to develop the links among

fractions, decimals, and percents

and solve problems involving their use.”

https://www.nctm.org/News-and-Calendar/Messages-from-the-President/Archive/Skip-Fennell/Fractions-Are-Foundational/

I don’t know about you, but I can’t agree more!

On the other hand, teachers often find it difficult to spend time explaining and reviewing rational number concepts secondary to a push to cover all material in a particular math curriculum. Are there ways to do this?

Solution? Provide students with review throughout the year via morning work and bell ringers. Add 4 – 6 questions to a math review page or test. Geometry problems such as finding perimeter and area are also ways to incorporate fractions. If the geometry problems include only whole numbers, have students change whole numbers to mixed numbers.

What about students who forget how to solve these problems? Provide a binder or posters with examples for solving rational number problems. If the student needs more assistance, pair the student with a student who is confident with the task.

In addition, you may be interested in the following resource that can be used for middle school students to review fraction operations.

FRACTION Operations BUNDLE: Mini-Lessons, Puzzles, Worksheets, Posters
Click on the link below.

https://www.teacherspayteachers.com/Product/FRACTION-Operations-BUNDLE-Mini-Lessons-Puzzles-Worksheets-Posters-9667771

#2 – Fractions: Math Concepts Before Middle School

Though middle school is often where the fraction, decimal and percent concepts become overwhelming for students, it is ideal to consider what happens in grades before middle school.

Do the students receive adequate instruction in fraction foundations? Are teachers given the support, additional training, and materials to teach fractions and other related concepts.?

Do students understand concepts related to adding, subtracting, multiplying, and dividing? Are they able to name and order rational numbers on a number line from 0 to 1?

These considerations are paramount in preparing students for success with fractions, decimals, and percentages. By providing support for teachers and students in grades before middle school, students entering middle school will be more successful with rational numbers.

#3 – Fractions: College Math

You see it every year. Students graduate from high school. Some of those students make plans to attend college. They submit scores for required entrance exams or take placement tests. Scores on these exams and tests indicate if the student must take a remedial course. So how many high school students are required to take remedial math courses?

According to the newspaper, The Tennessean, 46 percent of students in Tennessee, who graduated from high school and entered college for the school year, 2016-2017, were required to take remedial courses in math. That is almost half of high school graduates. That is a lot! Now let’s compare. Thirty-three percent of students were required to take remedial reading. This is still a very high number.

Likewise, in the following research article, Underprepared College Students’ Understanding of and Misconceptions with Fractions, Hea-Jin Lee 1* and Irina Boyadzhiev 1 explored student misconceptions of fractions. The study included 22 college students enrolled in a remedial math course.

The study demonstrated that students lacked the awareness of fraction definitions, such as least common denominator and least common multiple. The lack of these skills contributed to errors observed in fraction calculations.

Conclusion

Student challenges with rational numbers, (fractions, decimals, and percents), present a challenge at all levels of education. Yet, these concepts are so important for student success in math. Administrators of the school systems in grades K-12 must be encouraged to learn how they can help to increase teacher support and student success in math.

Looking for More Materials?

Click the button below to view more of my educational resources!

Learn More